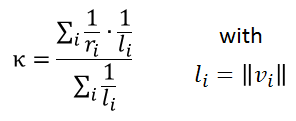Users:Structural Optimization/Response Functions/SurfaceCurvature
Contents |
General Description
Short Info
In the field of structural optimization it is often necessary to apply a constraining of surface curvature in order to maintain manufacturing constraints. To this purpose, Carat++ provides an estimation tool to approximate the mean curvature at a surface node.
Estimation of nodal curvature
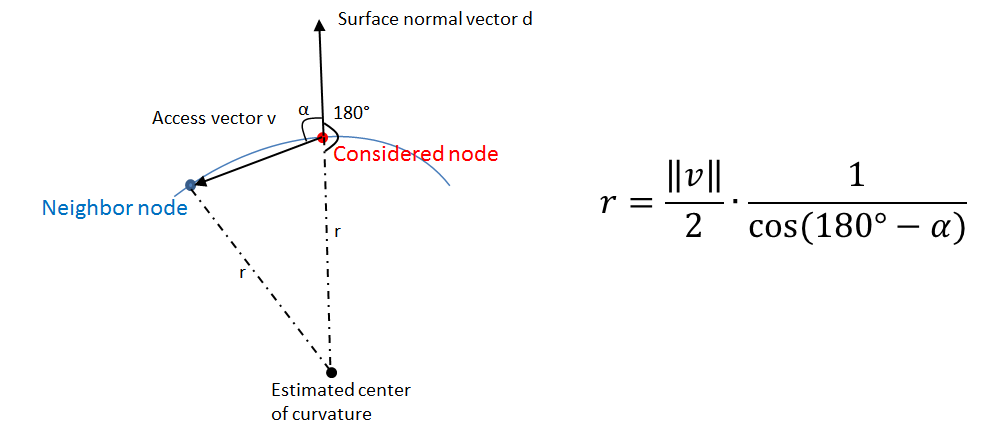
The nodal curvature is estimated using the surface normal vector and access vectors to the surrounding nodes. The estimation is based on a local spherical approximation of the geometry (see sketch below). So the radius of curvature r can be computed within the isosceles triangle.
Having computed this approximated radius of curvature for each surrounding node, the mean curvature of the surface is estimated by a weighted sum over all surrounding nodes i, whereat vi is the access vector to the ith node.
Kreisselmeier-Steinhauser weighting
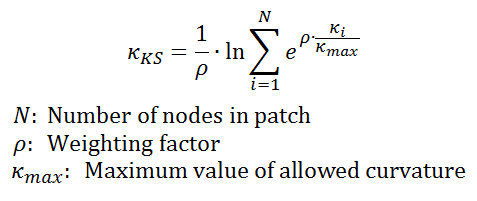
Instead of constraining each single nodal curvature, Carat++ provides a technique to control the curvature within bigger element patches. To this purpose a Kreisselmeier-Steinhauser weighting is used.
Via the weighting parameter ρ the user can control to which extend exceedings of the maximum allowable curvature are weighted.
Input Parameters
| Block headline | ||
| Parameter | Values, Default(*) | Description |
|---|---|---|
| OPT-RESPONSE_FCT | int : CURVATURE_PATCH_KS | Function ID and response function type. |
| Common compulsory parameters | ||
| ETA | real | Finite difference disturbance for sensitivity analysis |
| GRAD | DIRECT, ADJOINT | Method of gradient computation |
| SA | GLOBAL_FD, SEMI_ANALYTIC, EXACT_SEMI_ANALYTIC, ANALYTIC | Method of derivative computations inside sensitivity analysis |
| FDA | FOREWARD, CENTRAL, BACKWARD | Method of finite difference approximation (if neccessary for the chosen sensitivity analysis method) |
| DESVAR | OPT-VAR vector of integers | Design variables that are considered in the sensitivity analysis of this response function |
| Common optional parameters | ||
| WEIGHT | real, 1.0* | The weighting factor for this response function in multi-objective optimization |
| ANALYSIS | PC-ANALYSIS int | ID of the underlying analysis |
| Specific parameters | ||
| NODES | ND-SET int | Node set that includes all nodes this response function yields onto. |
| PATCH_SIZE | int | Size of patches (number of element rows) into which the NODES is sub-divided. |
| RHO | real | KS-weighting factor. |
| Common Compulsory Parameters for Constraints | ||
| Parameter | Values, Default(*) | Description |
| REL_LIMIT | real | Relative limit for constraint, depending on the actual value. |
| ABS_LIMIT | real | Absolute limit for constraint. Only one limit can be defined for a constraint. |
| CONSTRAINT_TYPE | INEQUALITY_LT, INEQUALITY_GT, EQUALITY | Type of constraint |
| Common Optional Parameters for Constraints | ||
| REL_TOLERANCE | real, 0* | Upper relative limit until which an inactive constraint is concidered as an active one |
| LAMBDA_ABS_MAX | real, 1/cepsilon* | Upper limit for lagrangian multiplier |
Example of a Complete Input Block
OPT-RESPONSE_FCT 1 : CURVATURE_PATCH_KS WEIGHT=1.0 ETA=1e-06 GRAD=ADJOINT SA= SEMI_ANALYTIC FDA=FOREWARD DESVAR=OPT-VAR 1 ! -- specific parameters NODES=ND-SET 1 PATCH_SIZE = 5 RHO = 15 ! -- constraint parameters ABS_LIMIT = 0.25 ! KAPPA_MAX REL_TOLERANCE = 0.1 CONSTRAINT_TYPE = INEQUALITY_LT LAMBDA_ABS_MAX = 40
A complete test example
Model description
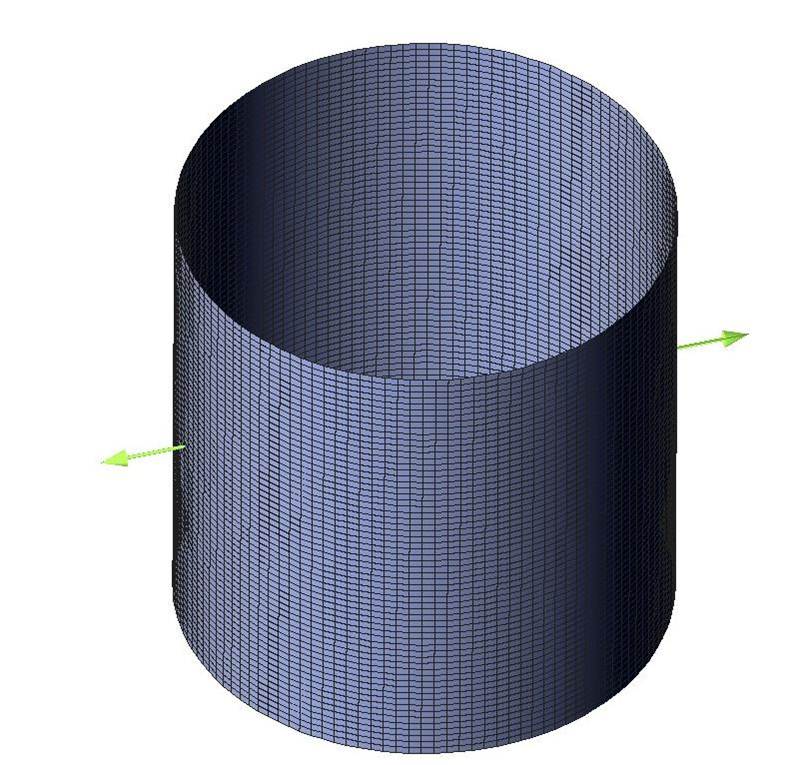
The considered test example is the optimization of a pinched cylinder applying a curvature limitation.
The following animation illustrates the eigenmodes of the plate.
Input File
Here the full input file can be downloaded.
Documented Results
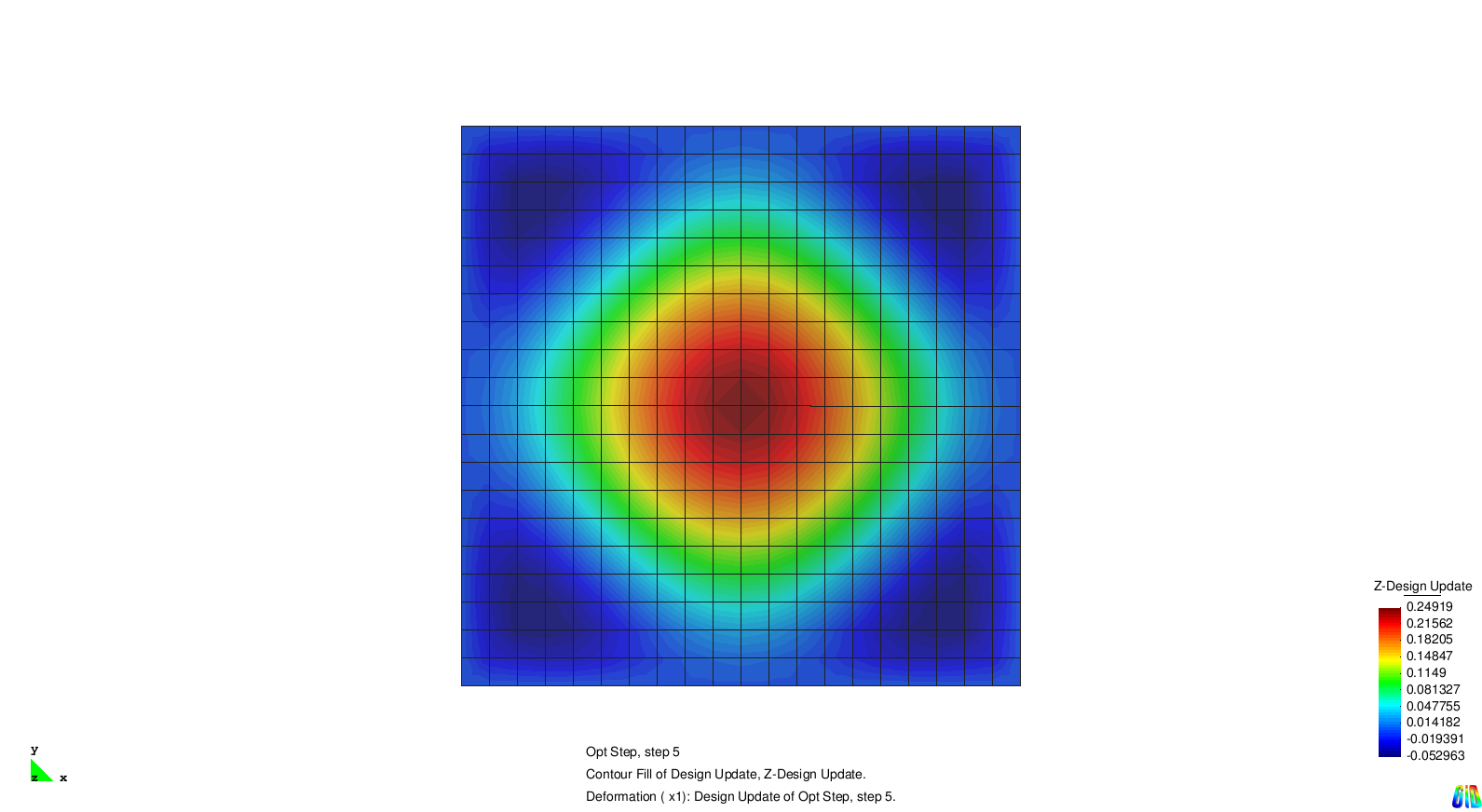
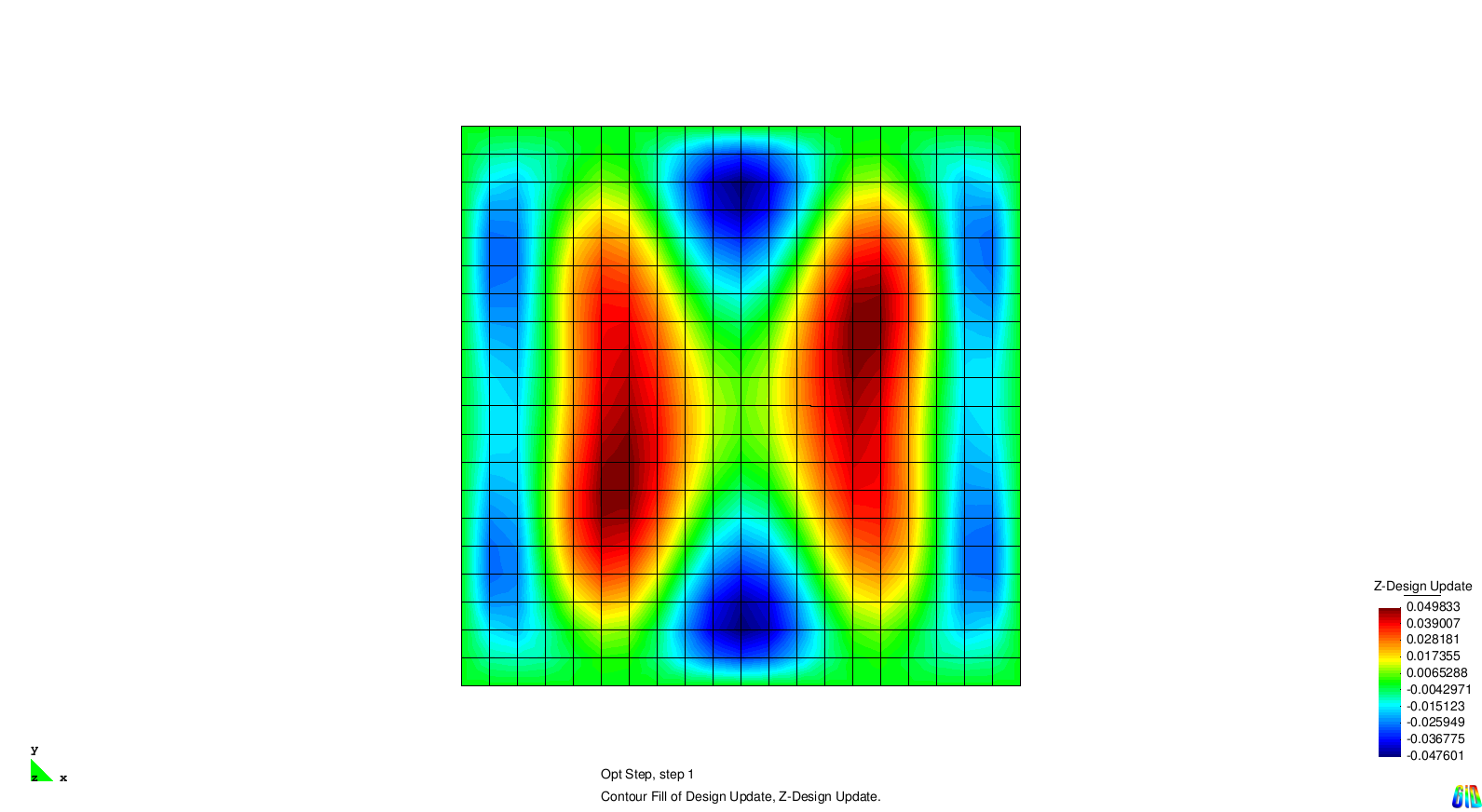
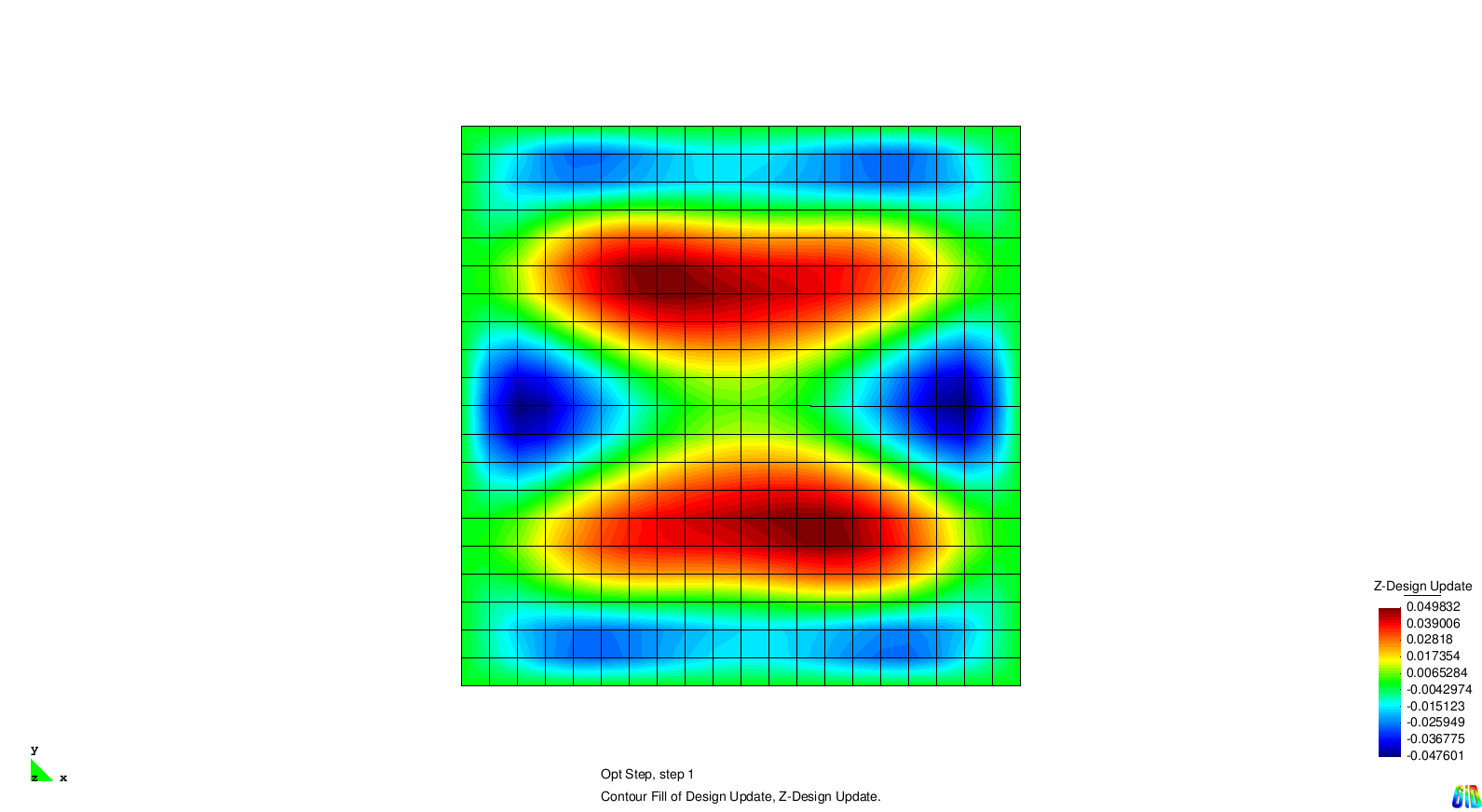
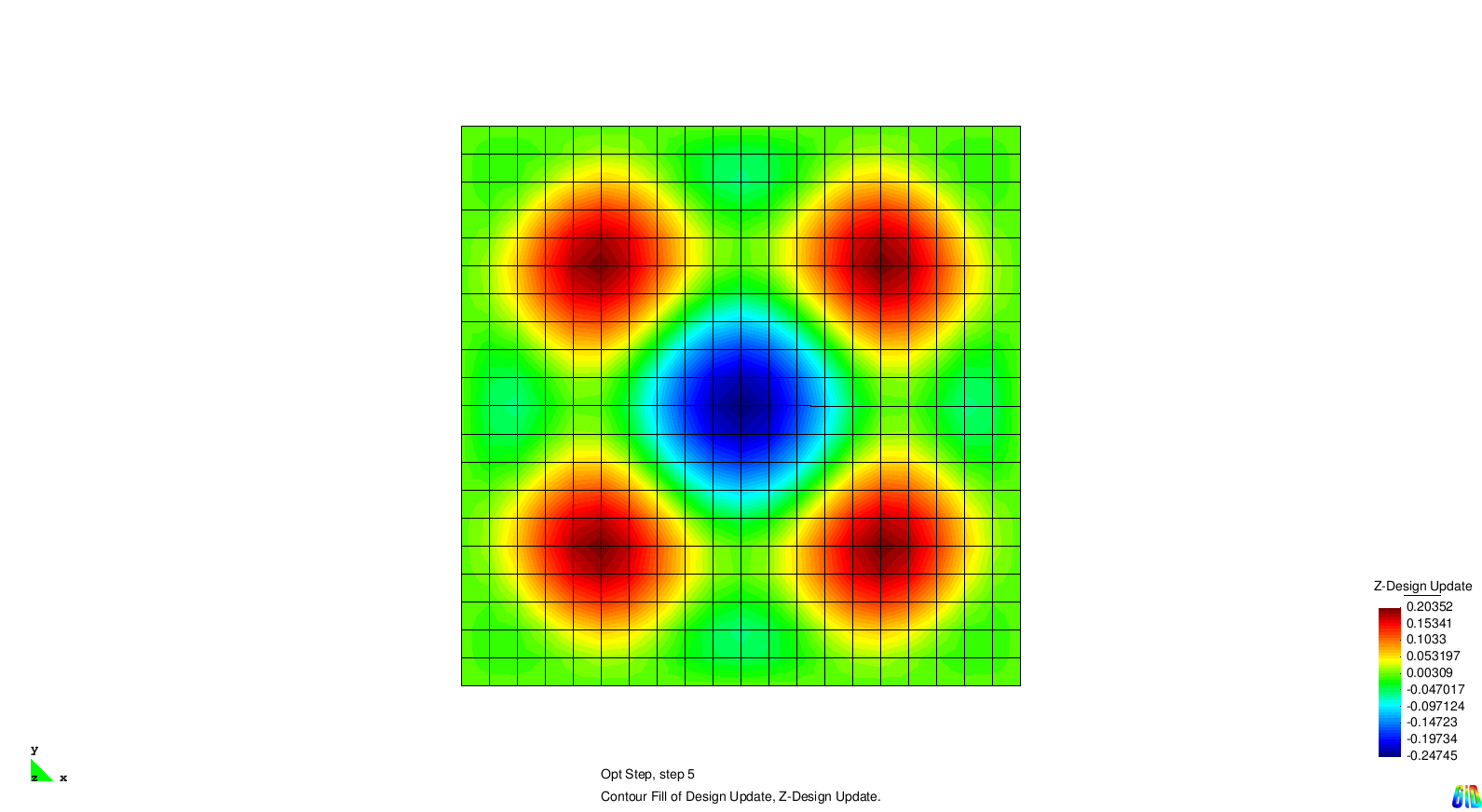
The results show the expected behaviour. Each solution generates stiffness towards a certain eigenmode. As the modes two and three are symmetric it is not surprising that the design updates are symmetric, too.
Optimization result w.r.t. 1st eigenmode
Optimization result w.r.t. 2nd eigenmode
Optimization result w.r.t. 3rd eigenmode
Optimization result w.r.t. 4th eigenmode
| Whos here now: Members 0 Guests 0 Bots & Crawlers 1 |
